Has to do with triangle, saw of cosine and sin, etc. I have to draw a polygon with 6-10 sides, and provide enough info for someone to be required to find 3 or more missing angles and 3 or more missing sides. Solve and find the area of the polygon.
Solution:
We’ll try the case of a six-sided polygon and will show that if we provide 3 angles, 3 sides and 5 spokes, we can expect someone to figure out the size of the three unknown angles and the lengths of the three unknown sides.
Here’s an example of a six-sided polygon, an irregular hexagon:
As you can see, this is an irregular shape – it’s not a regular hexagon where the sides and angles are of the same size.
Let’s now draw a central point and spokes, where you know the length of each spoke and all but one of the angles between the spokes:

You can calculate the unknown angle (we can call it “F”) because we know that all the angles need to add up to 360° (F = 360 – A – B – C – D – E). Since we know the length of two sides and one angle of each triangle that is formed (i.e. side-angle-side, or SAS for short), one can calculate the unknown side of each triangle using the Law of Cosines. Are you familiar with the Law of cosines and how it applies to an SAS case? You can find a lot of explanations online, but we can help you with this if you’re not able to find anything that you find easy to understand.
Now, let’s assume we know the length of three of the sides. If we know the length of the spokes and three of the sides (a, b and d shown below), then we only need to know two of the angles between the spokes (since the angles A, B, and D can be calculated using the Law of cosines) :

Now, let’s assume that we’re given two of the angles between the sides of the polyhedron (X and Y, shown below). In this case, we don’t need any of the angles between the spokes (E and C can be calculated using the Law of Sines, since this is a side-side-angle case, or SSA for short):

If we know one more angle (Z, shown below), then we can give up having to know one of the spokes, since we can calculate the length of the “unknown spoke” – shown with a dashed line – using the Law of Cosines (which applies because this is a side-angle-side case, or SAS for short):
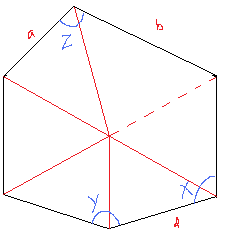
As you can see from the above diagram, if we provide three angles (X, Y and Z) and three sides (a, b and c) and 5 spokes of an irregular hexagon, we can expect someone to figure out the size of the unknown angles and the lengths of the unknown sides.
There are many ways to tackle this type of problem . we’ve shown another example below.
Here’s another approach.
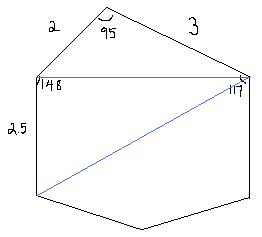
First, let’s provide some information on some of the sides and angles:

As you can see, we have indicated the lengths of three out of the six sides, and three out of the six angles (the diagram is not drawn to scale).
Now let’s draw a couple of lines as follows:
We can find the length of the blue line opposite the 95° angle by recognizing that we know the length of two sides and the angle between them (side-angle-side, or SAS for short). To find using the unknown side, we =an use cosine rule a² = b² + c² – 2bc cos(A), where A is the angle that we know (in this case 95°).
So we can re-write a² = b² + c² – 2bc cos(A) as follows:
a² = 9 + 4 – 12 cos(95°) = 13 – 12*(-0.087155) =13+ 1.0458689 = 14. 0458689, so a = 3.75.

We can now figure out the angle B using the fact that =² = a² + c² – 2ac cos(B): 9= 14. 0458689 + 4 – 2*3.75*2*cos(B).
We can now solve for cos(B) = 0 .6031
Using a lookup table or a scientific calculator we can see that B = 52.39.
Since we know that the angles of a triangle add up to 180°, we know A+B+C=180°. We know that A = 95 and B = 52.39, so C must equal =2.61. Now that we know that shape and size of the triangle, we can calculate its area. The area equals half the base times the height. The base is 3.75 and we can calculate the height by taking the sin of B and multiplying it by c =height =sin(0.6031)*2 = 0.792183*2 = 1.5844). Therefore the area of this triangle (with angles A, B and C) is 0.5*3.75*1.5844 = 2.9709.
We can now tackle the sides, angles and area of the next triangle, where one of the sides is equal to 2.5, and the other is 3.75:

We know that the angle between these two sides is equal to 95.61, since B = 52.39 and the sum of the two angles equals 148.
So now we have a similar problem, where we know the length of two sides and the size of the angle that they form (side-angle-side, or SAS). We can solve this just like we solved the problem above.
Once we have solved this second triangle, we’re Left with a quadrilateral for which we know only one side (d) and one angle (X):

There are too many unknowns to solve this, so we’ll need to provide a little more information so that someone can find length of the remaining sides and the size of the remaining angles.
One way to solve this is to break up the remaining area into smaller triangles, and provide information on some of the segments and angles:

As you can see from the above, we have created four new triangles, three of which are right angles. If we know p, q and r and the angle P, we can calculate the size and shape of each of the four triangles.
Posted in 11th Grade, Homework Answers, Precalculus.
This entry was posted on Thursday, July 22nd, 2010 at 9:00 am and is filed under 11th Grade, Homework Answers, Precalculus. You can follow any responses to this entry through the RSS 2.0 feed. You can leave a response, or trackback from your own site.